Calculus 1, part 2 of 2: Derivatives with applications
Differential calculus in one variable: theory and applications for optimisation, approximations, and plotting functions
4.95 (39 reviews)
1,409
students
56 hours
content
Oct 2024
last update
$79.99
regular price
What you will learn
How to solve problems concerning derivatives of real-valued functions of 1 variable (illustrated with 330 solved problems) and why these methods work.
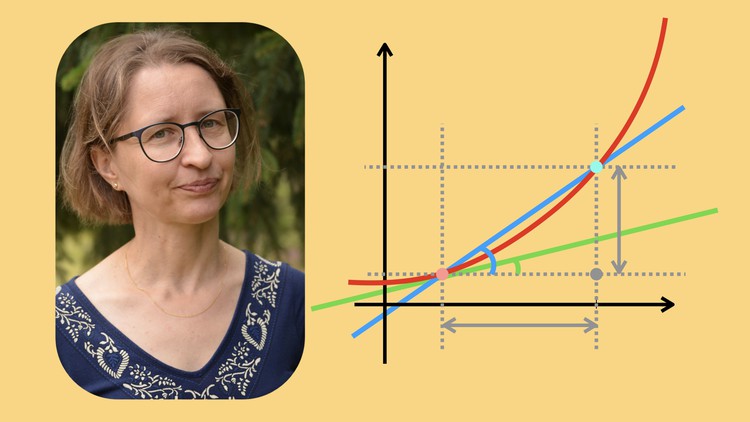
Definition of derivatives of real-valued functions of one real variable, with a geometrical interpretation and many illustrations.
Write equations of tangent lines to graphs of functions.
Derive the formulas for the derivatives of basic elementary functions.
Prove, apply, and illustrate the formulas for computing derivatives: the Sum Rule, the Product Rule, the Scaling Rule, the Quotient and Reciprocal Rule.
Prove and apply the Chain Rule; recognise the situations in which this rule should be applied and draw diagrams helping in the computations.
Use the Chain Rule in problem solving with related rates.
Use derivatives for solving optimisation problems.
Understand the connection between the signs of derivatives and the monotonicity of functions; apply first- and second-derivative tests.
Understand the connection between the second derivative and the local shape of graphs (convexity, concavity, inflection points).
Determine and classify stationary (critical) points for differentiable functions.
Use derivatives as help in plotting real-valued functions of one real variable.
Main theorems of Differential Calculus: Fermat's Theorem, Mean Value Theorems (Lagrange, Cauchy), Rolle's Theorem, and Darboux Property.
Formulate, prove, illustrate with examples, apply, and explain the importance of the assumptions in main theorems of Differential Calculus.
Formulate and prove l'Hospital's rule; apply it for computing limits of indeterminate forms; algebraical tricks to adapt the rule for various situations.
Higher order derivatives; an intro to Taylor / Maclaurin polynomials and their applications for approximations and for limits (more in Calculus 2).
Classes of functions: C^0, C^1, ... , C^∞; connections between these classes, and examples of their members.
Implicit differentiation with some illustrations showing horizontal and vertical tangent lines to implicit curves.
Logarithmic differentiation: when and how to use it.
A sneak peek into some future applications of derivatives.
Screenshots




5552258
udemy ID
9/11/2023
course created date
3/15/2024
course indexed date
Bot
course submited by